Amir R. Khoei
(To download a complete list of Publications click here)
|
Research interests |
|
Computational Nano-mechanics Computational plasticity (FEM, X_FEM and Meshless methods) Computational Fracture Mechanics Computational Geomechanics Large deformation analysis Error estimation and adaptivity for plasticity problems Contact and frictional modelling Strain localization analysis |
|
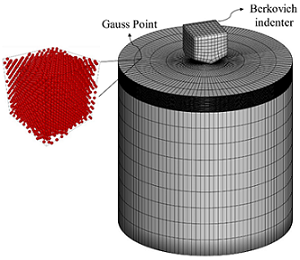
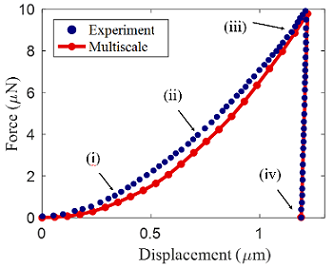
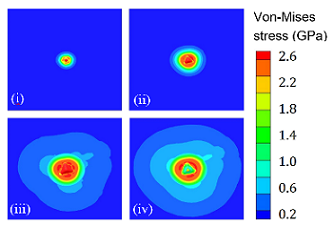
Modeling the nano-indentation test on the pure aluminum substrate with different crystal orientations; (a) the multiscale model, (b) a comparison of the multiscale result and experimental data, (c) the von-Mises stress contours on the surface of the aluminum substrate (See: International Journal of Mechanical Sciences, 108897, 2024). |
Computational Nano-Mechanics The purpose of my research activities in computational nano-mechanics is to investigate the characteristics of the material at nano-scale. The current research is based on the Molecular Dynamics simulations and the non-linear finite element simulations. Molecular Dynamics simulation is used as a standard tool in computational nano-mechanics. The research is conducted by developing a Molecular Dynamics software which is able to simulate multi-particle systems in canonical and micro-canonical ensembles. Furthermore, a machine learning-based multiscale approach is developed for modeling the crystalline nano-structures. To this end, a computational atomistic-continuum multiscale framework is developed based on the machine learning (ML) architecture to capture the nonlinear behavior of nano-crystalline structures. The dataset of the ML process is derived from analyzing the atomistic representative volume element (RVE) through the molecular dynamics method under various deformation paths. The multilayer perceptron neural network approach (MLP–ANN) is employed in the optimization algorithm of the ML process to train the atomistic dataset. It is shown that the proposed ML-based method enhances the multiscale analysis to capture the nonlinear behavior of nano-crystalline structures without computational complexities associated with conventional multiscale methods. The results highlight that the proposed multiscale framework can be utilized as a constitutive model for the large-scale analysis with a great accuracy and unique efficiency.
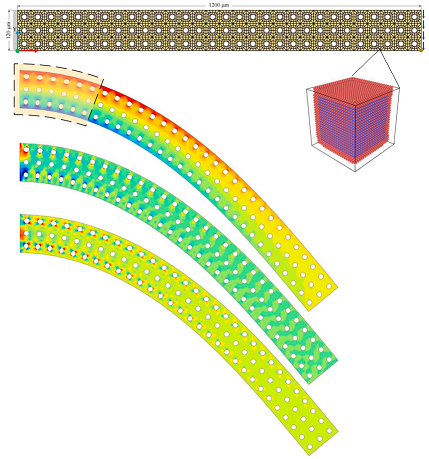
Multiscale modeling of a perforated cantilever micro-beam in bending (See: International Journal of Mechanical Sciences, 239, 107858, 2023). |
|
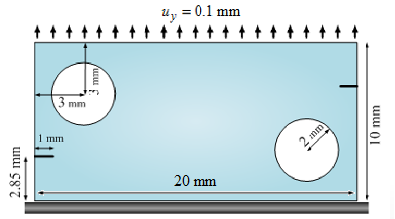
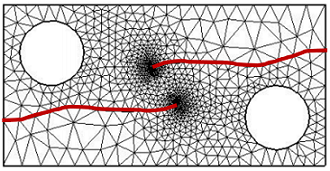
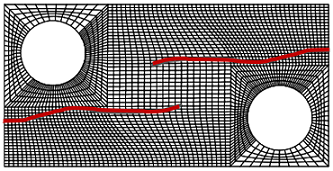
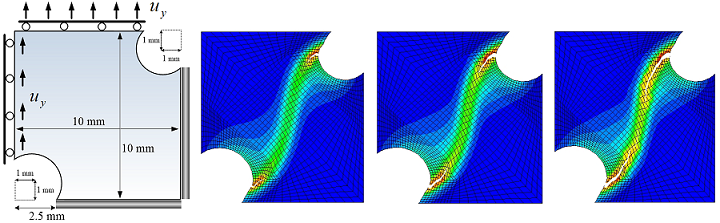
The crack trajectory for a plate with two holes; a) Geometry of the plate, b) Adaptive FEM method, C) Extended–FEM technique, d) The von-Mises stress contour (see A.R. Khoei, Extended Finite Element Method, Theory and Applications, John Wiley, 2015) |
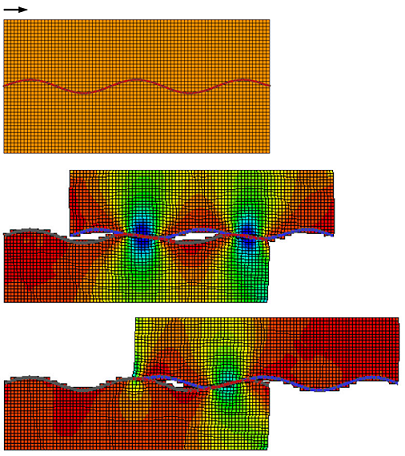
Computational Fracture Mechanics A comprehensive research work has been performed on fracture mechanics with special regards to crack growth and crack propagation in the brittle, cohesive and ductile materials. The numerical simulations are based on the adaptive FEM analysis and the X-FEM technique, which have been verified by the results of experimental tests. The method of adaptive finite element procedure is used to refine the mesh at the crack tip region based on the estimated error. The simulation of crack propagation was performed in 2D and 3D problems for both the elastic crack growth and the damage-plastic crack propagation. The other technique is based on the extended-FEM method, which is a state-of-the-art computational approach in FEM, and eliminates the necessity of using mesh generators in the analysis of continuum mechanics problems involving any kind of interfaces, such as bi-materials, contact problems, and fractured media. Moreover, a dynamic large deformation X-FEM method is proposed for modeling the full process of dynamic ductile fracture based on a nonlocal damage visco-plasticity model. The effect of inertia is modeled using an explicit central difference scheme which is enhanced through the use of mass lumping, reduced integration with hourglass control, and numerical damping. The material nonlinearity and the flow stress dependency on strain rate, hardening and temperature are modeled with the Johnson–Cook visco-plastic model. The micro void nucleation, growth and coalescence are modeled macroscopically with an isotropic damage model. The localization phenomenon due to the damage and thermal softening is suppressed by using the visco-plastic regularization in combination with a nonlocal visco-plastic model. The large deformation and large strain formulations are implemented within the X-FEM framework to model the macro crack discontinuities using an updated Lagrangian approach. Crack propagation and crack direction criteria are applied and the issues relating to the combination of X-FEM with the damage visco-plasticity model are addressed.
An enriched–FEM modeling of dynamic ductile fracture problems with a nonlocal damage–viscoplasticity model for a double notch problem (see Finite Elements in Analysis and Design, 99, 49-67, 2015) |
|
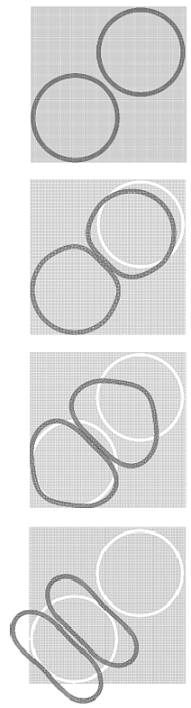
The contact–impact of two elastic rings; The deformed configurations at various time steps (see: Computer Methods in Applied Mechanics and Engineering, 269, 198-221, 2014) |
Computational Contact Mechanics The main area of research in computational solid mechanics encompasses large deformations, plasticity behaviour and contact problems for various solid mechanics including: metal forming applications and shear band localization problems. Both classical and Cosserat continuum mechanics have been employed in the framework of FEM analysis, adaptive FEM simulation, meshless technique, X-FEM modeling, and ALE formulation. Recent achievements in computational modeling of large plastic deformations are based on a three-invariant cap plasticity model and an enriched arbitrary Lagrangian-Eulerian finite element technique. A generalized cap plasticity with the isotropic-kinematic hardening rule is developed within the framework of an enriched arbitrary Lagrangian-Eulerian FE formulation. An enriched finite element method is implemented based on the extended FEM technique to capture the arbitrary interfaces independent of element boundaries. The process is accomplished by performing a splitting operator to separate the material (Lagrangian) phase from convective (Eulerian) phase, and partitioning the Lagrangian and relocated meshes with some sub-quadrilaterals whose Gauss points are used for integration of the domain of elements. The enriched ALE-FEM technique is applied to simulate the friction between two bodies by imposing the contact constraints and modifying the contact properties of frictional slip through the node-to-surface contact algorithm in the concept of penalty and augmented-Lagrange approaches.
Large deformation contact modeling of a block with a curved interface problem based on the phantom-node X-FEM technique (see: Computational Mechanics, 61, 449–469, 2018) |
|
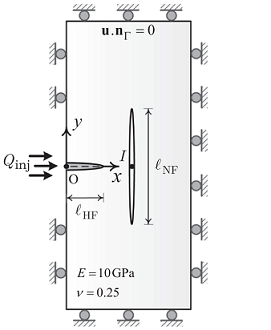
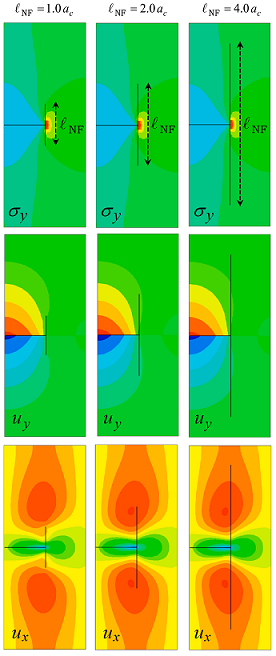
An enriched–FEM modeling of the hydro-fracture approaching a pre-existing natural fault together with the contours of vertical stress, vertical deformation and horizontal deformation at the intersection of hydro-fracture with the fully-cemented natural fault (Engineering Fracture Mechanics, 212, 269-290, 2019)
|
Computational Geomechanics Research activities in computational geotechniques have been developed based on the FEM and X-FEM modeling of deforming porous medium interacting with the flow of two immiscible wetting and non-wetting pore fluids. The governing equations are derived for unsaturated soils, such as the earth and rockfill dams, within the framework of the generalized Biot’s theory. An integrated software environment is designed for multi-disciplinary computational modeling of geotechnical problems, called SUT-DAM (Advances Engineering Software, 37, 728-753, 2006). The SUT-DAM is designed in both popularity and functionality with the development of user-friendly pre- and post-processing software. In SUT-DAM, a numerical model is developed based on a Lagrangian finite element formulation for large deformation dynamic analysis of saturated and partially saturated soils. An adaptive FEM strategy is used by employing an error estimator, adaptive mesh refinement, and data transfer operator. The SUT-DAM supports different yield criteria, including the classical and advanced constitutive models, such as the Pastor-Zienkiewicz and cap plasticity models. Furthermore, an enriched finite element method is developed to model the interaction between the hydraulically-driven fracture and the naturally-cemented fault within the porous medium. The inflow and continuity equations of the fluid phase are solved throughout the discontinuities in conjunction with the momentum balance equation of the bulk using a sequential manner based on the staggered Newton algorithm. The frictional contact behavior along the overlapped zone of the naturally-cemented fault is modeled through the extended–FEM penalty scheme. The effect of cementation bond along the natural fault is incorporated employing a modified Coulomb law to derive the cementation tractions. Various interaction scenarios are recognized based on an approach accounting for the effect of fracturing fluid diversion into the intersected fracture network.
A nine-spot well pattern with a hydraulically fractured production and different natural fracture pattern; Contours of water saturation in fractured reservoir after 7 years of water injection (Advances in Water Resources, 94, 510-528, 2016) |
|
Software Design |
|
|
|
SUT_DAM (version 4.0) An integrated software environment for geotechnical engineering For more information about the software see the following paper: A.R. Khoei, S.A. Gharehbaghi, A.R. Azami and A.R. Tabarraie, ‘SUT-DAM: An integrated software environment for multi-disciplinary geotechnical engineering’, Advances Engineering Software, 37, 728-753, 2006. |